Graduate School Research - PhD
PhD work
Introduction
Feynman, Schwinger, and Tomonaga were awarded the 1965 Nobel Prize in physics for their contributions to quantum electrodynamics. To date this theory–QED–is the most precise theory ever developed. It has the precision of predicting the distance between you and the moon with an error of measuring from your chin or the top of your head. However, Feynman also lamented
It seems that very little physical intuition has yet been developed in this subject. In nearly every case we are reduced to computing exactly the coefficient of some specific term. We hvave no way to get a general ideas of the result to be expected…We have been computing terms like a blind man exploring a new room, but soon we must develop some concept of this room as a whole, and to have some general idea of what is contained in it. As a specific challenge, is there any method of computing the anomalous moment of the electron which, on first rough approximation, gives a fair approximation to the \(\alpha\) term…
Just as Feynman said, we have this impressively succesful theory with zero intuition of it. And: more than 70 years later, we still have no idea what any of it means and how to relate to it. So, in an attempt to better understand the inner workings of QED, we have approached the quantum realm from a classical perspective through the notion of a polarizable medium.
In the standard model, this polarizable medium can be viewed as pair generation (particle-antiparticle dipoles) which emerge from the vacuum. Dirac even argued at the first Solvay Conference that the observed electronic charge of the electron/positron must be smaller than the ‘‘bare’’ charge by an amount of \(\approx 1/137\), the fine structure constant, and this difference between the observed charge and bare charge is likely caused by pair creation and annihilation of cirtual electron-positron pairs. As Sakurai states:
'’This situation is rather analogous to that of a charge placed in a dielectric material; the effective charge in a polarized medium is the original charge divided by \(\epsilon\), where \(\epsilon\) is the dielectric constant. In other words, because of virtual electron-positron pairs the vacuum behaves like a polarizable medium.’’
The proposed effect for hydrogen-like atoms is a splitting of the \(2s_{\frac{1}{2}}-2p_{\frac{1}{2}}\) states. The correction term needed for this splitting–called Zitterbewegung or the ‘‘Darwin term’’–can be explained as the atomic electron annihilating with the positron of the virtual pair. So the question remains, does the classical polarizable medium model account for the \(2s_{\frac{1}{2}}-2p_{\frac{1}{2}}\) state splitting?
Results
One natural consequence of this classical approach is a classical renormalization of point charges. Just like the more advanced field theory approach, this classical approach also resolves the self-energy paradox. Here, we demonstrate how the polarized vacuum provides a correction to the classical Coulomb potential at short distance but asymptotically reduces to the Coulomb potential for distances larger than the classical electron radius.
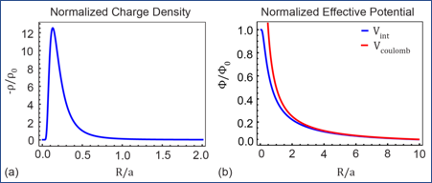
(a)Total charge density according to the renormalized solution through the notion of a polarizable vacuum. (b) The new effective potential \(\Phi_{VP}/\Phi_0 = 1-exp(-a/2r)\) is compared to the electrostatic Coloumb potential \(\Phi_C = q/4\pi\varepsilon_0 r\).
Applying this general solution to the electron yields
(a)Total charge density according to the renormalized solution through the notion of a polarizable vacuum. (b) The new effective potential \(\Phi_{VP}/\Phi_0 = 1-exp(-a/2r)\) is compared to the electrostatic Coloumb potential \(\Phi_C = q/4\pi\varepsilon_0 r\).
To address the splitting the \(2s_{\frac{1}{2}}-2p_{\frac{1}{2}}\) states we analyzed the positronium and hydrogen atoms. Here we only provide results for the positronium atom. Our psoitrium model shows first order corrections equivalent to the so-called Darwin term, Zitterbewegung. This is the first classical theorem that can successfully account for pair annihilation, as is commonly encountered with PET imaging.
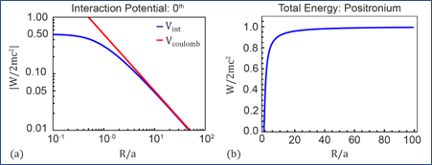Plotted is the interaction potential \(V_int\). At large distances the interaction potential converges to the Coulomb potential. (b) The total energy is plotted as a function of separation distance. As the two particles approach (\(R/a \rightarrow 0\)) each other and the separation goes to zero, the total energy goes to zero at the origin. This is synonymous with annihilation. For the first time, we have a self-consistent description of fundamental particle interactions without infinities and including pair annihilation.
Future Work
Size
While this toy model shows promise for providing a classical intuition for QED phenomena more work is needed. For example, the experimentally determined radius of the electron is approximately zero. The polarizable medium model also shows an expected radius of \(<r> = 0\). However, the proton and neutron, which are comprised of three point charges (e.g. up and down quarks), have measurable size. So we are left to address this effective size discrepancy.
The Anomalous Magnetic Moment of the Electron
Only QED has been successful at account for the anomalous magnetic moment of the electron. For this toy model to have significant impact in developing intuition of QED it must account for the anomalous moment.
Hydrogen Revisited
Previous results for the hydrogen atom using the polarizable medium model were developed assuming the proton to be point-like. While results properly account for the Darwin term a more complete model is available if we use a 4-particle model.
