Grad School Projects - Photonic Bose-Einstein Condensate

23 Mar 2014 in Posts

This work was for Statistical Mechanics

Authors

• Neal Blackman
• Raj Vinnakota
• Brandon Touchet

Introduction

In thermodynamics and statistical mechanics a Bose-Einstein Condensate (BEC) is the phase transition from a classical gas to a quantum degenerated gas. BECs form when a gas reaches a critical temperature, \(T_c~(n^(2/3) h^2)/(mk_B )\) , or critical density, ρ_c. The critical density can be roughly defined as a density such that the de Broglie thermal wavelength of the particles is greater than the inter-particle separation, which gives rise to a thermal wavelength of \(λ_(th_c )~1/n\), where n is the number of particles in the system. When \(λ_(th_c)\) is reached the wave-functions of the particles overlap and form a “quantum liquid”, which behaves as a single particle. For a gas of integer spin (Bosons) the particles fall into their ground state, exhibiting coherence.

Table 1 shows a sequential outline of breakthroughs involving the BEC. Eric Cornell and Carl Weinman, at the University of Colorado at Boulder, produced the first true BEC in 1995. All previous attempts to form BECs were more closely exhibiting superfluidity, zero viscosity, which arises at the lambda transition. The lambda transition is a location on a heat capacity versus temperature graph, \(C_v\) vs \(T\), where the heat capacity spikes at one point and the plot resembles the Greek letter “\(λ\)”. For gases such as He the corresponding critical temperature for the lambda transition is 2.17K. The difference between superfluidity and condensation is that superfluidity deals with transport properties and requires interacting particles while the latter corresponds to equilibrium properties and is better achieved with an idea gas, non-interacting.

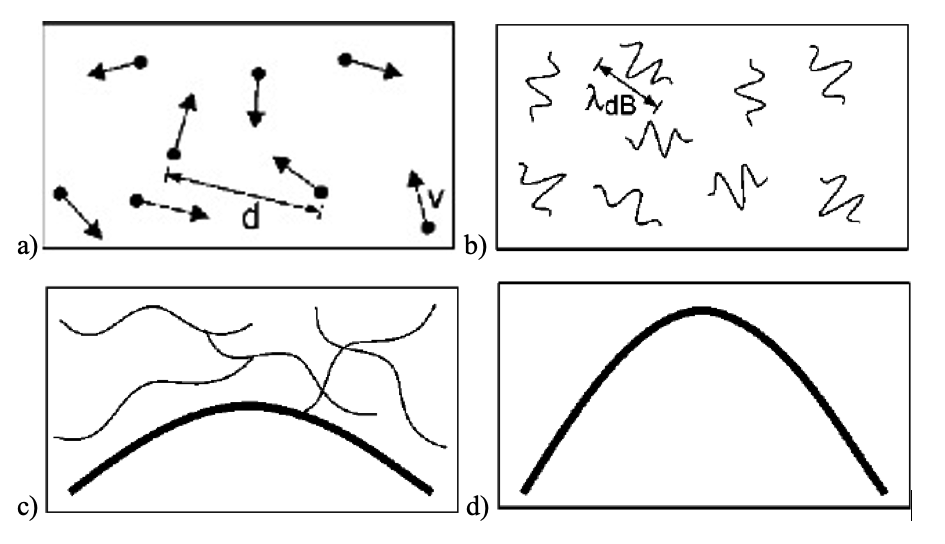Weakly interacting gas at high temperature. b) The atoms can be regarded as wave packets with an extension of their de Broglie wavelength \(λ_db\). c) At \(T<T_c\) the matter waves of each individual atom overlaps and \(λ_db\) is comparable to the inter-atomic distance and approaching the BEC state. d) At \(T<T_c\) the sea of wave packets disappears and behaves like single matter wave giving rise to the BEC.

Until 2010 all previous BECs were achieved with ultra-cold atomic gases, solid-state quasiparticles, exciton-polaritons, and magnons. In the present literature review (Schmitt et al. [1]) a novel BEC is attained by confining photons within a dye-fill microcavity, where the photons are allowed to reach thermal equilibrium by repeated absorption-emission dye molecules. As if a BEC of photons is not novel enough, Schmitt et al. form their BEC at room temperature (\(~300K\)) which is approximately ten orders of magnitude hotter than the BEC produced by Cornell and Weinman. The dye in this system acts as a heat bath and a particle reservoir due to the frequent interactions of the electrons with photons, which leads to molecular electronic excitation. These excitations allow for fluctuations of the particle number around a (controllable) average value— a situation that, for large reservoirs, could be well described using a grand-canonical statistical ensemble.

Photonic BEC

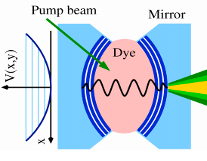
Photons have spin 1 and could theoretically be used to form a BEC, but, thus far, the scientific community has not found a way to force a photon gas into thermal equilibrium. Schmitt et al. proposed and provided extensive proof of their methodology for achieving a photonic BEC. The general experimental setup used to form a “super-photon”, a Bose-Einstein Condensate of photons, consists of two slightly curved mirrors (radius of curvature,R ~ 1m, reflectivity >99.99%) separated by a distance of 1.5 μm, forming a micro-resonator. The shape of the micro-cavity provides a harmonic potential in which the lowest energy state will form in the center of the cavity, along the axis of the micro-cavity (See Figure 2). A collection of dye molecules is placed within the confines of the micro-resonator. The dye molecules are universally known for their unique absorption/emission spectra— they are the things that give our clothes their color.

Depiction of the harmonic potential provided on the left. A depiction of the micro-cavity provided on the right. [3]

Previous works by Schmitt et al. attempted to form a BEC of exciton-polaritons. Excitons are merely just the bound state of an electron and an electron hole, which are attracted via the Coulomb force, and exciton-polaritons are qausi-particles arising from strong coupling of excitons and photons. A BEC of exciton-polaritons can arise from a thermalization process—the reaching of thermal equilibrium by particle interactions, shown to conserve average particle number—induced by inter-particle interactions between the exciton-polaritons and photons. Unfortunately for the group, frequent collisions between dye molecules and the solvent resulted in de-coherence of transverse mode. Coherence is a defining quality of a BEC and for a proposed ensemble to lose or fail to exhibit coherence, by definition, is no longer or was never a BEC. The characteristic mirror spacing, D_0, being in the micrometer regime, coupled with specific dye molecules can result in the resonator being populated by photons of a single longitudinal mode number. Subsequently, the photon gas becomes two-dimensional and the dispersion relation resembles that of a massive particle. The associated effective mass of the photons is many orders of magnitude smaller than that of a hydrogen atom. Such a small effective mass provides a dispersion relation that is quadratic, as one expects to find with massive particles. By requiring that the thermal de Broglie wavelength, λ_th, of the effective mass of the photon be much larger than the cavity spacing (satisfying the λ_(th_c ) condition) we can guarantee that there exists only one longitudinal mode, to a very good approximation. The longitudinal resonance condition is given by k_r (r)=qπ/(D_0-2(R-√(R^2-r^2 )) , where r is the distance from the optical axis, D0 is the relative spacing between the two mirrors on the optical axis, and R is the radius of curvature. Since there is only one longitudinal mode, then there exist two quantized transversal modes (TEM00 being the lowest allowed mode). The ensemble of dye molecules can absorb and emit photons, but the emitted photons are of lower frequencies (longer wavelengths) than the absorbed photons (See Figure 1). When the cavity thermalizes the rovibrational dye manifold the cavity modes fulfill the Kennard-Stepanov relation: \(α(ω)/β(ω) =w_↑/w_↓ e^(-ℏ(ω-ω_0)/k_B T)\) where α(ω) is the absorption spectrium, β(ω) is the emission spectrum, ω_0 is the zero-phonon line, and w_↑ and w_↓ are statistical weights related to the rovibrational states. The weights can be found to be \[w_↓=∑_(α∈S_0)▒e^(ε_α/k_B T) and w_↑=∑_(β∈S_1)▒e^(ε_β/k_B T) , where ε_β+ℏω_0=ε_α+ℏω.\]

Photon thermalization can occur if the photon lifetime is longer than the time for the micro-cavity to reach thermal equilibrium. Collisions between the dye molecules are on the order of a femtosecond (fs, 10-15s) while thermalization of the rovibrational states is on the order of picoseconds (ps, 10-9s). Fortunately, the average photon lifetime is on the order of a nanosecond. A continuous in-flow of photons (pump pulses ≈150ns) is supplied to compensate losses due to unrestricted optical modes, finite mirror reflectivity, and non-radiative decay. The repeated absorption and emission of the photons by the dye molecules acts as a heat bath and particle reservoir. By altering the number of dye molecules and their associated chemical composition the ensemble conditions will change, and subsequently alter the ensemble type from micro-canonical to grand-canonical or visa versa. The photon condensation will occur when the phase space density exceeds a value near unity. Subsequently, based on the experimental setup described above, condensation is achieved at room temperature. The grand-canonical ensemble forms with a sufficiently large particle number in addition to satisfying the Kennard-Stepanov-Relation.

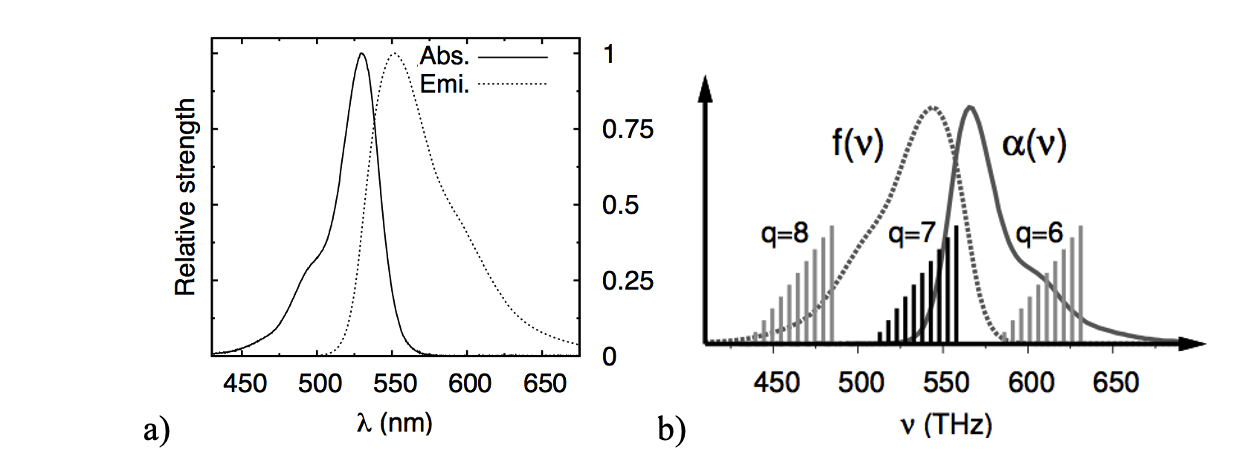a) Shows the emission and absorption spectrum of the dye molecules. This spectrum is used to tune the micro-cavity for the most appropriate cut-off frequency.[2] b) Shows the same relationship with the longitudinal and transverse modes, where the vertical bars represent the degeneracy of the transverse modes. [3]

Figure 3 provides the emission and absorption spectrum for the dye molecules. By tuning the micro-cavity they can sufficiently space there is approximately one longitudinal mode within the cavity. This is useful to tune the micro-cavity to exclude all unnecessary radiation. If there is overlap there will be excess radiation; subsequently, the signal will not follow a Boltzmann distribution of transversally excited modes, for the given equilibrium temperature. As shown in Figure 4, the spectra adequately follows the theory with the correct cutoff wavelength.

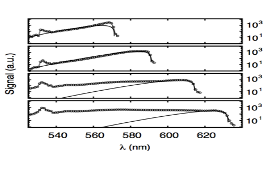Shows Intensity of the cavity for different cutoff wavelengths. The dots represent the data. The solid line represents the theoretical Boltzmann distribution for transversally excited modes. [1]

Figure 5 shows proof of the BEC. As seen in Figure 5a(a) the fluctuations verify grand-canonical ensemble conditions for a BEC, where the fluctuations are on the order of the number of particles in the ensemble, N. As the number of particles in the condensed state, n, increases the fluctuations approach the average value of the number of particles in the condensed state, n0. In other words, as n0 approaches N we should achieve canonical ensemble conditions and the fluctuations should cease.

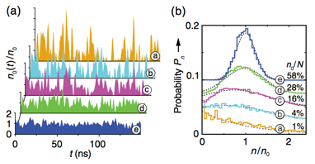Shows Intensity of the cavity for different cutoff wavelengths. The dots represent the data. The solid line represents the theoretical Boltzmann distribution for transversally excited modes. [1]

References

[1] Phys. Rev. Lett. 112, 030401 (2014) [5 pages]

[2] arXiv:1210.7707 [cond-mat.quant-gas]

[3] Nature 468, pgs 545–548, (25 November 2010)